Work done for IITJEE | CBSC 11th | ICSC

Introduction to work
In our daily life work has many different meanings, for example, Ravi is working in a factory. the machine is in working order.
In physics, however, the term work has a specific meaning.in Physics, work is always associated with a force and displacement. we know that for work to be done, the force must act through a distance consider, suppose, a person holding a weight at distance ‘s’ off the floor. In everyday use, we might say that the man is doing work but in our scientific definition, no work is done by a force acting on a stationary object. we could eliminate the effort of holding the weight by simply attaching the string to some object and the weight could be supported with no help from us.

Work done:

There are three methods of finding work done:-

• Work done by a constant force.
• Work done by a variable force.
• Work is done by the area under the F-S graph.

(i) Work done by a constant force

Let us first consider the simple case of a constant force acting on a body. Let also assume that the body moves in a straight line in the direction of the force. In this case, we define the work done by the force on the body as the product of the magnitude of force ‘F’ and the distance ‘s’ through which the body moves.

That is the work ‘w’ is given by:

\(W=F\times S\)

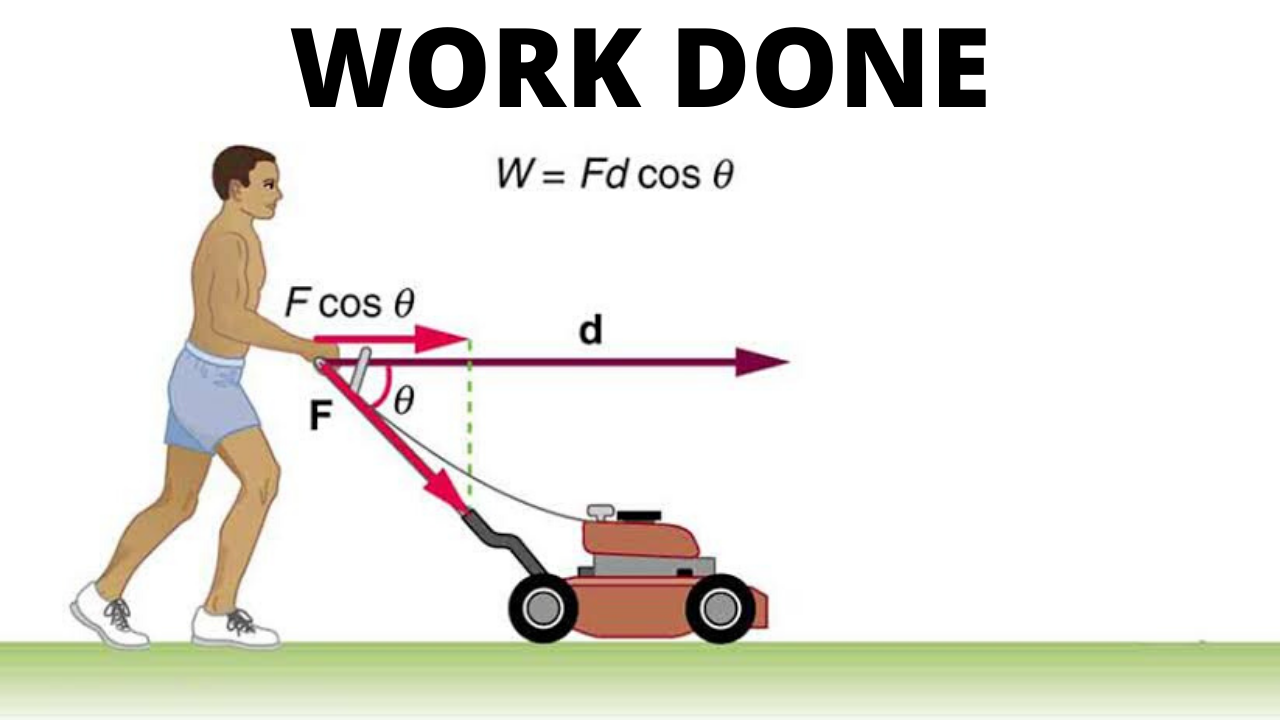
On the other hand, in a situation, when the constant force ‘F’ does not act along the same direction as the displacement ‘S’ of the body the component of force along the displacement ‘S’ is effective in doing work.
Thus, in this case, work done by the constant force ‘F’ is given by;

W=(a component of force along with the displacement) * (displacement)

\(\begin{aligned}W=F\cos \left( \theta \right) \times S\\ W=\overrightarrow {F}\cdot \overrightarrow {S}\end{aligned}\)

Work can be positive, negative or even zero also depending on the angle between the force vector ‘F’ and displacement vector ‘S’. work done by a force is zero when the angle is equal to 90, it is positive when theta is less than 90 and negative when theta is greater than 90.

For example, when a person lifts a body the work done by the lifting force is positive as the angle is equal to zero. But work done by the force of gravity is negative as the angle is equal to 180. similar work done by the centripetal force is always zero, as the angle is equal to 90.

Work depend on the frame of reference. with the change of frame of reference initial force does not change while displacement may change so, the work done by the force will be different in a different frame.

For example, if a person is pushing a box inside a moving train when work done is seen for a frame of reference of the train is (F*S) while as seen from the ground it is

\(\overrightarrow {F}\cdot \left( \overrightarrow {S}+\overrightarrow {S_{0}}\right)\)

here \( S_{0}\) is the displacement of the train relative to the ground.
Suppose a body is displaced from point A to point B then

\(\overrightarrow {S}=\overrightarrow {r_{B}}-\overrightarrow {r_{A}}\)